Chapter 15 Equilibria of Other Reaction Classes
15.4 Coupled Equilibria
Learning Objectives
By the end of this section, you will be able to:
- Describe examples of systems involving two (or more) coupled chemical equilibria
- Calculate reactant and product concentrations for coupled equilibrium systems
As discussed in preceding chapters on equilibrium, coupled equilibria involve two or more separate chemical reactions that share one or more reactants or products. This section of this chapter will address solubility equilibria coupled with acid-base and complex-formation reactions.
An environmentally relevant example illustrating the coupling of solubility and acid-base equilibria is the impact of ocean acidification on the health of the ocean’s coral reefs. These reefs are built upon skeletons of sparingly soluble calcium carbonate excreted by colonies of corals (small marine invertebrates). The relevant dissolution equilibrium is
Rising concentrations of atmospheric carbon dioxide contribute to an increased acidity of ocean waters due to the dissolution, hydrolysis, and acid ionization of carbon dioxide:
The equilibrium constant for this net reaction is much greater than the Ksp for calcium carbonate, indicating its solubility is markedly increased in acidic solutions. As rising carbon dioxide levels in the atmosphere increase the acidity of ocean waters, the calcium carbonate skeletons of coral reefs become more prone to dissolution and subsequently less healthy (Figure 15.7).
Link to Learning
Learn more about ocean acidification and how it affects other marine creatures.
This site has detailed information about how ocean acidification specifically affects coral reefs.
The dramatic increase in solubility with increasing acidity described above for calcium carbonate is typical of salts containing basic anions (e.g., carbonate, fluoride, hydroxide, sulfide). Another familiar example is the formation of dental cavities in tooth enamel. The major mineral component of enamel is calcium hydroxyapatite (Figure 15.8), a sparingly soluble ionic compound whose dissolution equilibrium is
This compound dissolved to yield two different basic ions: triprotic phosphate ions
and monoprotic hydroxide ions:
Of the two basic productions, the hydroxide is, of course, by far the stronger base (it’s the strongest base that can exist in aqueous solution), and so it is the dominant factor providing the compound an acid-dependent solubility. Dental cavities form when the acid waste of bacteria growing on the surface of teeth hastens the dissolution of tooth enamel by reacting completely with the strong base hydroxide, shifting the hydroxyapatite solubility equilibrium to the right. Some toothpastes and mouth rinses contain added NaF or SnF2 that make enamel more acid resistant by replacing the strong base hydroxide with the weak base fluoride:
The weak base fluoride ion reacts only partially with the bacterial acid waste, resulting in a less extensive shift in the solubility equilibrium and an increased resistance to acid dissolution. See the Chemistry in Everyday Life feature on the role of fluoride in preventing tooth decay for more information.
Chemistry in Everyday Life
Role of Fluoride in Preventing Tooth Decay
As we saw previously, fluoride ions help protect our teeth by reacting with hydroxylapatite to form fluorapatite, Ca5(PO4)3F. Since it lacks a hydroxide ion, fluorapatite is more resistant to attacks by acids in our mouths and is thus less soluble, protecting our teeth. Scientists discovered that naturally fluorinated water could be beneficial to your teeth, and so it became common practice to add fluoride to drinking water. Toothpastes and mouthwashes also contain amounts of fluoride (Figure 15.9).
Unfortunately, excess fluoride can negate its advantages. Natural sources of drinking water in various parts of the world have varying concentrations of fluoride, and places where that concentration is high are prone to certain health risks when there is no other source of drinking water. The most serious side effect of excess fluoride is the bone disease, skeletal fluorosis. When excess fluoride is in the body, it can cause the joints to stiffen and the bones to thicken. It can severely impact mobility and can negatively affect the thyroid gland. Skeletal fluorosis is a condition that over 2.7 million people suffer from across the world. So while fluoride can protect our teeth from decay, the US Environmental Protection Agency sets a maximum level of 4 ppm (4 mg/L) of fluoride in drinking water in the US. Fluoride levels in water are not regulated in all countries, so fluorosis is a problem in areas with high levels of fluoride in the groundwater.
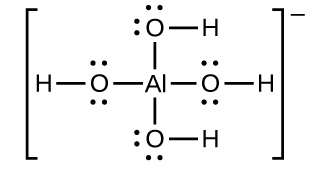
The solubility of ionic compounds may also be increased when dissolution is coupled to the formation of a complex ion. For example, aluminum hydroxide dissolves in a solution of sodium hydroxide or another strong base because of the formation of the complex ion Al(OH)4−.
The equations for the dissolution of aluminum hydroxide, the formation of the complex ion, and the combined (net) equation are shown below. As indicated by the relatively large value of K for the net reaction, coupling complex formation with dissolution drastically increases the solubility of Al(OH)3.
Example 15.17 – Increased Solubility in Acidic Solutions
Compute and compare the molar solublities for aluminum hydroxide, Al(OH)3, dissolved in (a) pure water and (b) a buffer containing 0.100 M acetic acid and 0.100 M sodium acetate.
Solution
(a) The molar solubility of aluminum hydroxide in water is computed considering the dissolution equilibrium only as demonstrated in several previous examples:
| Al(OH)3(s) | ⇌ | Al3+(aq) | + | 3 OH−(aq) | |
| I (M) | — | 0 | 0 | ||
| C (M) | (−x) | +x | +3x | ||
| E (M) | — | x | 3x |
[latex]K_{sp} = [Al^{3+}] \, [OH^-]^3[/latex]
(b) The concentration of hydroxide ion of the buffered solution is conveniently calculated by the Henderson-Hasselbalch equation:
[latex]\text {pH} = \text pK_a + \log \frac {[CH_3COO^-]}{[CH_3COOH]} = 4.74 + \log \frac {0.100 \: M}{0.100 \: M} = 4.74[/latex]
At this pH, the concentration of hydroxide ion is
Check Your Learning
Click here to see a walkthrough for these problems!
The pH of the buffered solution is first calculated using the Henderson-Hasselbalch equation:
[latex]\text {pH} = \text pK_a + \log \frac {[HCOO^-]}{[HCOOH]} = 3.74 + \log \frac {0.100 \: M}{0.100 \: M} = 3.74[/latex]
At this pH, the concentration of hydroxide ion is
Example 15.18 – Multiple Equilibria
Unexposed silver halides are removed from photographic film when they react with sodium thiosulfate (Na2S2O3, called hypo) to form the complex ion Ag(S2O3)23− (Kf = 4.7 × 1013).
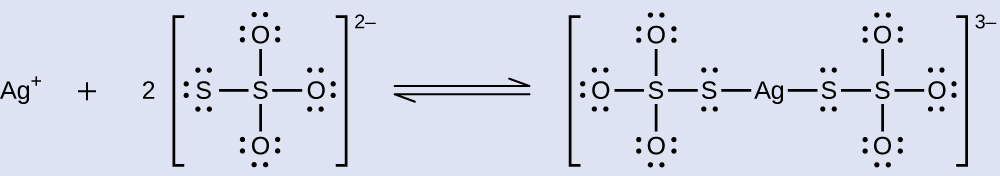
What mass of Na2S2O3 is required to prepare 1.00 L of a solution that will dissolve 1.00 g of AgBr by the formation of Ag(S2O3)23−?
Solution
Two equilibria are involved when silver bromide dissolves in an aqueous thiosulfate solution containing the S2O32− ion:
dissolution: AgBr(s) ⇌ Ag+(aq) + Br−(aq) Ksp = 5.0 × 10−13
complexation: Ag+(aq) + 2 S2O32−(aq) ⇌ Ag(S2O3)23−(aq) Kf = 4.7 × 1013
[latex][/latex]
Combining these two equilibrium equations yields
AgBr(s) + 2 S2O32−(aq) ⇌ Ag(S2O3)23−(aq) + Br−(aq)
[latex]K = \frac {[Ag(S_2O_3)_2^{3-}] \, [Br^-]}{[S_2O_3^{2-}]^2} = K_{sp} \, K_f = (5.0 \times 10^{-13}) \, (4.7 \times 10^{13}) = 24[/latex]
The initial concentration of silver resulting from dissolution of 1.00 g of AgBr in 1.00 L of solution is
Mass of Na2S2O3 required to yield 0.00110 M S2O32-:
[latex]0.0011 \: \frac {\cancel {mol \: S_2O_3^{2-}}}{1.00 \: L} \: \left( \frac {1 \cancel {mol \: Na_2S_2O_3}}{1 \cancel {mol \: S_2O_3^{2-}}} \right) \: \left( \frac {158.11 \: g \: Na_2S_2O_3}{1 \cancel {mol \: Na_2S_2O_3}} \right) = 0.17 \: g \: Na_2S_2O_3[/latex]
The mass of Na2S2O3 required to dissolve 1.00 g of AgBr in 1.00 L of water is thus 1.68 g + 0.17 g = 1.85 g
Check Your Learning
AgCl(s), silver chloride, has a very low solubility: AgCl(s) ⇌ Ag+(aq) + Cl−(aq), Ksp = 1.6 × 10–10.
Adding ammonia significantly increases the solubility of AgCl because a complex ion is formed: Ag+(aq) + 2 NH3(aq) ⇌ Ag(NH3)2+(aq), Kf = 1.7 × 107.
What mass of NH3 is required to prepare 1.00 L of solution that will dissolve 2.00 g of AgCl by formation of Ag(NH3)2+?
Click here for the solution and a walkthrough!
Two equilibria are involved when silver chloride dissolves in an aqueous solution containing ammonia (NH3) ion:
dissolution: AgCls) ⇌ Ag+(aq) + Cl−(aq) Ksp = 1.6 × 10−10
complexation: Ag+(aq) + 2 NH3(aq) ⇌ Ag(NH3)2+(aq) Kf = 1.7 × 107
[latex][/latex]
Combining these two equilibrium equations yields
AgCl(s) + 2 NH3(aq) ⇌ Ag(NH3)2+(aq) + Cl−(aq)
[latex]K = \frac {[Ag(NH_3)_2^{+}] \, [Cl^-]}{[NH_3]^2} = K_{sp} \, K_f = (1.6 \times 10^{-10}) \, (1.7 \times 10^{7}) = 0.0027[/latex]
The initial concentration of silver resulting from dissolution of 2.00 g of AgCl in 1.00 L of solution is
[latex]0.269 \: \frac {\cancel {mol \: NH_3}}{1.00 \: L} \: \left( \frac {17.031 \: g \: NH_3}{1 \cancel {mol \: NH_3}} \right) = 4.58 \: g \: NH_3[/latex]
The mass of NH3 required to dissolve 1.00 g of AgCl in 1.00 L of water is thus 0.477 g + 4.58 g = 5.06 g