17.1  Oxidation-Reduction Chemistry

Nicole Bouvier-Brown; Saori Shiraki; J. Ryan Hunt; Emily Jarvis

Chapter 17 Electrochemistry

17.1 Oxidation-Reduction Chemistry

Learning Objectives

By the end of this section, you will be able to:

Describe defining traits of redox chemistry
Calculate oxidation states
Identify the oxidant and reductant of a redox reaction
Balance chemical equations for redox reactions using the half-reaction method

Oxidation-Reduction Reactions

Earth’s atmosphere contains about 20% molecular oxygen, O₂, a chemically reactive gas that plays an essential role in the metabolism of aerobic organisms and in many environmental processes that shape the world. The term oxidation was originally used to describe chemical reactions involving O₂, but its meaning has evolved to refer to a broad and important reaction class known as oxidation-reduction (redox) reactions. A few examples of such reactions will be used to develop a clear picture of this classification.

Some redox reactions involve the transfer of electrons between reactant species to yield ionic products, such as the reaction between sodium and chlorine to yield sodium chloride:

2 Na(s) + Cl₂(g) → 2 NaCl(s)

It is helpful to view the process with regard to each individual reactant; that is, to represent the fate of each reactant in the form of an equation called a half-reaction:

[latex][/latex]

2 Na(s) → 2 Na⁺(s) + 2 e⁻

Cl₂(g) + 2 e⁻ → 2 Cl(s)

These equations show that Na atoms lose electrons while Cl atoms (in the Cl₂ molecule) gain electrons, the “s” subscripts for the resulting ions signifying they are present in the form of a solid ionic compound. For redox reactions of this sort, the loss and gain of electrons define the complementary processes that occur:

[latex][/latex]

oxidation = loss of electrons

reduction = gain of electrons

[latex][/latex]

In this reaction, then, sodium is oxidized and chlorine undergoes reduction. Viewed from a more active perspective, sodium functions as a reducing agent (reductant), since it provides electrons to (or reduces) chlorine. Likewise, chlorine functions as an oxidizing agent (oxidant), as it effectively removes electrons from (oxidizes) sodium.

[latex][/latex]

reducing agent = species that is oxidized

oxidizing agent = species that is reduced

Some redox processes, however, do not involve the transfer of electrons. Consider, for example, a reaction similar to the one yielding NaCl:

[latex][/latex]

H₂(g) + Cl₂(g) → 2 HCl(g)

[latex][/latex]

The product of this reaction is a covalent compound, so transfer of electrons in the explicit sense is not involved. To clarify the similarity of this reaction to the previous one and permit an unambiguous definition of redox reactions, a property called oxidation number has been defined.

Oxidation Numbers

The oxidation number (or oxidation state) of an element in a compound is the charge its atoms would possess if the compound were ionic. The following guidelines are used to assign oxidation numbers to each element in a molecule or ion.

The oxidation number of an atom in an elemental substance is zero.
The oxidation number of a monatomic ion is equal to the ion’s charge.
Metals always have positive oxidation states.
1. Group 1 metals always have an oxidation state of +1
2. Group 2 metals always have an oxidation state of +2
Oxidation numbers for common nonmetals are usually assigned as follows:
1. Hydrogen: +1 when combined with nonmetals, −1 when combined with metals
2. Oxygen: −2 in most compounds, sometimes −1 (so-called peroxides, O₂²⁻), very rarely − 1/2 (so-called superoxides, O₂⁻), positive values when combined with F (values vary)
3. Halogens: −1 for F always, −1 for other halogens except when combined with oxygen or other halogens (positive oxidation numbers in these cases, varying values)
The sum of oxidation numbers for all atoms in a molecule or polyatomic ion equals the charge on the molecule or ion.

Note: The proper convention for reporting charge is to write the number first, followed by the sign (e.g., 2+), while oxidation number is written with the reversed sequence, sign followed by number (e.g., +2). This convention aims to emphasize the distinction between these two related properties.

Example 17.1 – Assigning Oxidation Numbers

Follow the guidelines in this section of the text to assign oxidation numbers to all the elements in the following species:

(a) H₂S

(b) SO₃²⁻

(c) Na₂SO₄

Solution

(a) According to guideline 4, the oxidation number for H is +1.

Using this oxidation number and the compound’s formula, guideline 5 may then be used to calculate the oxidation number for sulfur:

[latex][/latex]

[latex]\text{charge on} \ H_2S = 0 = \left( 2 \times (+1) \right) + \left( 1 \times x \right)[/latex]

[latex][/latex]

[latex]x = 0 - \left( 2 \times (+1) \right) = -2[/latex]

(b) Guideline 4 suggests the oxidation number for oxygen is −2.

Using this oxidation number and the ion’s formula, guideline 5 may then be used to calculate the oxidation number for sulfur:

[latex][/latex]

[latex]\text{charge on} \: SO_3^{2-} = -2 = \left( 3 \times (-2) \right) + \left( 1 \times x \right)[/latex]

[latex][/latex]

[latex]x = -2 - \left( 3 \times (-2) \right) = +4[/latex]

(c) For ionic compounds, it’s convenient to assign oxidation numbers for the cation and anion separately.

According to guideline 3, the oxidation number for sodium is +1.

Assuming the usual oxidation number for oxygen (−2 per guideline 3), the oxidation number for sulfur is calculated as directed by guideline 5:

[latex][/latex]

[latex]\text{charge on} \: SO_4^{2-} = -2 = \left( 4 \times (-2) \right) + \left( 1 \times x \right)[/latex]

[latex][/latex]

[latex]x = -2 - \left( 4 \times (-2) \right) = +6[/latex]

Check Your Learning

Click here for a walkthrough of these problems!

Question 1

According to guideline 3, the oxidation number for K is +1. According to guideline 4, the oxidation number of O is probably -2.

Guideline 5 may then be used to calculate the oxidation number for nitrogen:

[latex][/latex]

[latex]\text{charge on} \ KNO_3 = 0 = \left( 1 \times (+1) \right) + \left( 1 \times x \right) + \left( 3 \times (-2) \right)[/latex]

[latex][/latex]

[latex]x = 0 - 1 + 6 = +5[/latex]

Question 2

Because hydrogen is combined with a metal (Al), it exists as hydride in AlH₃ and has an oxidation number of -1. Metals like Al always have positive oxidation numbers.

Guideline 5 may then be used to calculate the oxidation number for aluminum:

[latex][/latex]

[latex]\text{charge on} \ AlH_3 = 0 = \left( 1 \times x \right) + \left( 3 \times (-1) \right)[/latex]

[latex][/latex]

[latex]x = 0 - 3 = +3[/latex]

Question 3

According to guideline 4, the oxidation number for H is +1.

Guideline 5 may then be used to calculate the oxidation number for nitrogen:

[latex][/latex]

[latex]\text{charge on} \ NH_4^+ = +1 = \left( 1 \times x \right) + \left( 4 \times (+1) \right)[/latex]

[latex][/latex]

[latex]x = +1 - 4 = -3[/latex]

Question 4

According to guideline 4, the oxidation number for H is +1 and the oxidation number of O is probably -2.

Guideline 5 may then be used to calculate the oxidation number for nitrogen:

[latex][/latex]

[latex]\text{charge on} \ H_2PO_4^- = -1 = \left( 2 \times (+1) \right) + \left( 1 \times x \right) + \left( 4 \times (-2) \right)[/latex]

[latex][/latex]

[latex]x = - 1 - 2 + 8 = +5[/latex]

Using the oxidation number concept, an all-inclusive definition of redox reaction has been established. Oxidation-reduction (redox) reactions are those in which one or more elements involved undergo a change in oxidation number. (While the vast majority of redox reactions involve changes in oxidation number for two or more elements, a few interesting exceptions to this rule do exist.) Definitions for the complementary processes of this reaction class are correspondingly revised as shown here:

[latex][/latex]

oxidation = increase in oxidation number

reduction = decrease in oxidation number

[latex][/latex]

Returning to the reactions used to introduce this topic, they may now both be identified as redox processes. In the reaction between sodium and chlorine to yield sodium chloride, sodium is oxidized (its oxidation number increases from 0 in Na to +1 in NaCl) and chlorine is reduced (its oxidation number decreases from 0 in Cl₂ to −1 in NaCl). In the reaction between molecular hydrogen and chlorine, hydrogen is oxidized (its oxidation number increases from 0 in H₂ to +1 in HCl) and chlorine is reduced (its oxidation number decreases from 0 in Cl₂ to −1 in HCl).

Several subclasses of redox reactions are recognized, including combustion reactions in which the reductant (also called a fuel) and oxidant (often, but not necessarily, molecular oxygen) react vigorously and produce significant amounts of heat, and often light, in the form of a flame. Solid rocket-fuel reactions are combustion processes. A typical propellant reaction in which solid aluminum is oxidized by ammonium perchlorate is represented by this equation:

10 Al(s) + 6 NH₄ClO₄(s) → 4 Al₂O₃(s) + 2 AlCl₃(s) + 12 H₂O(g) + 3 N₂(g)

[latex][/latex]

Link to Learning

Watch a brief video showing the test firing of a small-scale, prototype, hybrid rocket engine planned for use in the new Space Launch System being developed by NASA. The first engines firing at 3 s (green flame) use a liquid fuel/oxidant mixture, and the second, more powerful engines firing at 4 s (yellow flame) use a solid mixture.

Single-displacement (replacement) reactions are redox reactions in which an ion in solution is displaced (or replaced) via the oxidation of a metallic element. One common example of this type of reaction is the acid oxidation of certain metals:

[latex][/latex]

Zn(s) + 2 HCl(aq) → ZnCl₂(aq) + H₂(g)

Metallic elements may also be oxidized by solutions of other metal salts; for example:

[latex][/latex]

Cu(s) + 2 AgNO₃(aq) → Cu(NO₃)₂(aq) + 2 Ag(s)

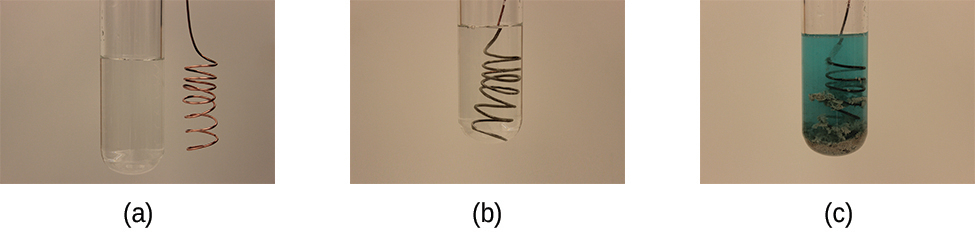
This reaction may be observed by placing copper wire in a solution containing a dissolved silver salt. Silver ions in solution are reduced to elemental silver at the surface of the copper wire, and the resulting Cu²⁺ ions dissolve in the solution to yield a characteristic blue color (Figure 17.2).

.

**Figure 17.2** (a) A copper wire is shown next to a solution containing silver(I) ions. (b) Displacement of dissolved silver ions by copper ions results in (c) accumulation of gray-colored silver metal on the wire and development of a blue color in the solution, due to dissolved copper ions. (credit: modification of work by Mark Ott)

Example 17.2 – Describing Redox Reactions

Identify which equations represent redox reactions.

For those reactions identified as redox, name the oxidant and reductant.

(a) ZnCO₃(s) → ZnO(s) + CO₂(g)

(b) 2 Ga(l) + 3 Br₂(l) → 2 GaBr₃(s)

(c) 2 H₂O₂(aq) → 2 H₂O(l) + O₂(g)

(d) BaCl₂(aq) + K₂SO₄(aq) → BaSO₄(s) + 2 KCl(aq)

(e) C₂H₄(g) + 3 O₂(g) → 2 CO₂(g) + 2 H₂O(l)

Solution

By definition, a redox reaction is a reaction where one or more elements undergo a change in oxidation number.

(a) This is not a redox reaction, since oxidation numbers remain unchanged for all elements.

(b) This is a redox reaction.

Gallium is oxidized, its oxidation number increasing from 0 in Ga(l) to +3 in GaBr₃(s). The reducing agent is Ga(l).
Bromine is reduced, its oxidation number decreasing from 0 in Br₂(l) to −1 in GaBr₃(s). The oxidizing agent is Br₂(l).

(c) This is a redox reaction. It is a particularly interesting process, as it involves the same element, oxygen, undergoing both oxidation and reduction (a so-called disproportionation reaction).

Oxygen is oxidized, its oxidation number increasing from −1 in H₂O₂(aq) to 0 in O₂(g).
Oxygen is also reduced, its oxidation number decreasing from −1 in H₂O₂(aq) to −2 in H₂O(l).

(d) This is not a redox reaction, since oxidation numbers remain unchanged for all elements.

(e) This is a redox reaction (combustion).

Carbon is oxidized, its oxidation number increasing from −2 in C₂H₄(g) to +4 in CO₂(g). The reducing agent (fuel) is C₂H₄(g).
Oxygen is reduced, its oxidation number decreasing from 0 in O₂(g) to −2 in H₂O(l). The oxidizing agent is O₂(g).

Check Your Learning

Balancing Redox Reactions via the Half-Reaction Method

Redox reactions that take place in aqueous media often involve water, hydronium ions, and hydroxide ions as reactants or products. Although these species are not oxidized or reduced, they do participate in chemical change in other ways (e.g., by providing the elements required to form oxyanions). Equations representing these reactions are sometimes very difficult to balance by inspection, so systematic approaches have been developed to assist in the process. One very useful approach is to use the method of half-reactions, which involves the following steps:

Write the two half-reactions representing the redox process.
Balance all elements except oxygen and hydrogen.
Balance oxygen atoms by adding H₂O molecules.
Balance hydrogen atoms by adding H⁺ ions.
Balance charge by adding electrons.
If necessary, multiply each half-reaction’s coefficients by the smallest possible integers to yield equal numbers of electrons in each.
Add the balanced half-reactions together and simplify by removing species that appear on both sides of the equation.
For reactions occurring in basic media (excess hydroxide ions), carry out these additional steps:
1. Add OH⁻ ions to both sides of the equation in numbers equal to the number of H⁺ ions.
2. On the side of the equation containing both H⁺ and OH⁻ ions, combine these ions to yield water molecules.
3. Simplify the equation by removing any redundant water molecules.
Finally, check to see that both the number of atoms and the total charges^[1] are balanced.

Example 17.3 – Balancing Redox Reactions in Acidic Solution

Write a balanced equation for the reaction between dichromate ion and iron (II) to yield iron (III) and chromium (III) in acidic solution.

Cr₂O₇²⁻ + Fe²⁺ → Cr³⁺ + Fe³⁺

Solution

Step 1. Write the two half-reactions. Each half-reaction will contain one reactant and one product with one element in common.

Fe²⁺ → Fe³⁺

Cr₂O₇²⁻ → Cr³⁺

Step 2. Balance all elements except oxygen and hydrogen.

The iron half-reaction is already balanced

Fe²⁺ → Fe³⁺

The chromium half-reaction shows two Cr atoms on the left and one Cr atom on the right. Changing the coefficient on the right side of the equation to 2 achieves balance with regard to Cr atoms.

Cr₂O₇²⁻ → 2 Cr³⁺

Step 3. Balance oxygen atoms by adding H₂O molecules.

The iron half-reaction does not contain O atoms.

Fe²⁺ → Fe³⁺

The chromium half-reaction shows seven O atoms on the left and none on the right, so seven water molecules are added to the right side.

Cr₂O₇²⁻ → 2 Cr³⁺ + 7 H₂O

Step 4. Balance hydrogen atoms by adding H⁺ions.

The iron half-reaction does not contain H atoms.

Fe²⁺ → Fe³⁺

The chromium half-reaction shows 14 H atoms on the right and none on the left, so 14 hydrogen ions are added to the left side.

Cr₂O₇²⁻ + 14 H⁺ → 2 Cr³⁺ + 7 H₂O

Step 5. Balance charge by adding electrons.

The iron half-reaction shows a total charge of 2+ on the left side (1 Fe²⁺ ion) and 3+ on the right side (1 Fe³⁺ ion). Adding one electron to the right side brings that side’s total charge to (3+) + (1−) = 2+, and charge balance is achieved.

Fe²⁺ → Fe³⁺ + e⁻

The chromium half-reaction shows a total charge of (1 × 2−) + (14 × 1+) = 12+ on the left side (1 Cr₂O₇²⁻ion and 14 H⁺ ions). The total charge on the right side is (2 × 3+) = 6 + (2 Cr³⁺ ions). Adding six electrons to the left side will bring that side’s total charge to (12+ + 6−) = 6+, and charge balance is achieved.

Cr₂O₇²⁻ + 14 H⁺ + 6 e⁻ → 2 Cr³⁺ + 7 H₂O

Step 6. Multiply the two half-reactions so the number of electrons in one reaction equals the number of electrons in the other reaction.

To be consistent with mass conservation, and the idea that redox reactions involve the transfer (not creation or destruction) of electrons, the iron half-reaction’s coefficient must be multiplied by 6.

6 Fe²⁺ → 6 Fe³⁺ + 6e⁻

Cr₂O₇²⁻ + 6 e− + 14 H⁺ → 2 Cr³⁺ + 7 H₂O

Step 7. Add the balanced half-reactions and cancel species that appear on both sides of the equation.

6 Fe²⁺ + Cr₂O₇²⁻ + 6 e⁻ + 14 H⁺ → 6 Fe³⁺ + 6 e⁻ + 2 Cr³⁺ + 7 H₂O

Only the six electrons are redundant species. Removing them from each side of the equation yields the simplified, balanced equation here:

6 Fe²⁺ + Cr₂O₇²⁻ + 6 e⁻ + 14 H⁺ → 6 Fe³⁺ + 6 e⁻ + 2 Cr³⁺ + 7 H₂O

[latex][/latex]

6 Fe²⁺ + Cr₂O₇²⁻ + 14 H⁺ → 6 Fe³⁺ + 2 Cr³⁺ + 7 H₂O

[latex][/latex]

A final check of atom and charge balance confirms the equation is balanced.

	Reactants	Products
Fe	6	6
Cr	2	2
O	7	7
H	14	14
charge	24+	24+

Check Your Learning

Write a balanced equation for the reaction between iron (II) and permanganate to yield iron (III) and manganese (II) in acidic solution.

Fe²⁺ + MnO₄^– → Fe³⁺ + Mn²⁺

Click here for the answer and a walkthrough!

Step 1. Write the two half-reactions. Each half-reaction will contain one reactant and one product with one element in common.

Fe²⁺ → Fe³⁺

MnO₄^– → Mn²⁺

Step 2. Balance all elements except oxygen and hydrogen.

The iron half-reaction is already balanced

Fe²⁺ → Fe³⁺

The manganese half-reaction is already balanced.

MnO₄^– → Mn²⁺

Step 3. Balance oxygen atoms by adding H₂O molecules.

The iron half-reaction does not contain O atoms.

Fe²⁺ → Fe³⁺

The manganese half-reaction shows four O atoms on the left and none on the right, so four water molecules are added to the right side.

MnO₄^– → Mn²⁺ + 4 H₂O

Step 4. Balance hydrogen atoms by adding H⁺ions.

The iron half-reaction does not contain H atoms.

Fe²⁺ → Fe³⁺

The manganese half-reaction shows 8 H atoms on the right and none on the left, so 8 hydrogen ions are added to the left side.

MnO₄^–+ 8 H⁺ → Mn²⁺ + 4 H₂O

Step 5. Balance charge by adding electrons.

The iron half-reaction shows a total charge of 2+ on the left side (1 Fe²⁺ ion) and 3+ on the right side (1 Fe³⁺ ion). Adding one electron to the right side brings that side’s total charge to (3+) + (1−) = 2+, and charge balance is achieved.

Fe²⁺ → Fe³⁺ + e⁻

The manganese half-reaction shows a total charge of (1 × 1−) + (8 × 1+) = 7+ on the left side. The total charge on the right side is 2+. Adding six electrons to the left side will bring that side’s total charge to (12+ + 6−) = 6+, and charge balance is achieved.

MnO₄^– + 8 H⁺ + 5e⁻ → Mn²⁺ + 4 H₂O

Step 6. Multiply the two half-reactions so the number of electrons in one reaction equals the number of electrons in the other reaction.

To be consistent with mass conservation, and the idea that redox reactions involve the transfer (not creation or destruction) of electrons, the iron half-reaction’s coefficient must be multiplied by 5.

5 Fe²⁺ → 5 Fe³⁺ + 5e⁻

MnO₄^– + 8 H⁺ + 5 e⁻ → Mn²⁺ + 4 H₂O

Step 7. Add the balanced half-reactions and cancel species that appear on both sides of the equation.

5 Fe²⁺ + MnO₄^– + 5 e⁻ + 8 H⁺ → 5 Fe³⁺ + 5 e⁻ + Mn²⁺ + 4 H₂O

Only the five electrons are redundant species. Removing them from each side of the equation yields the simplified, balanced equation here:

5 Fe²⁺ + MnO₄^– + 5 e⁻ + 8 H⁺ → 5 Fe³⁺ + 5 e⁻ + Mn²⁺ + 4 H₂O

[latex][/latex]

5 Fe²⁺ + MnO₄^– + 8 H⁺ → 5 Fe³⁺+ Mn²⁺ + 4 H₂O

[latex][/latex]

A final check of atom and charge balance confirms the equation is balanced.

Example 17.4 – Balancing Equations for Redox Reactions in Acidic Solutions

Write the balanced equation representing reaction between solid copper and nitric acid to yield aqueous copper(II) ions and nitrogen monoxide gas.

Solution

Step 1. Write the two half-reactions. Each half-reaction will contain one reactant and one product with one element in common.

oxidation: Cu(s) → Cu²⁺(aq)

reduction: HNO₃(aq) → NO(g)

Step 2. Balance all elements except oxygen and hydrogen.

The copper half-reaction is already balanced

Cu(s) → Cu²⁺(aq)

The nitric acid half-reaction is already balanced.

HNO₃(aq) → NO(g)

Step 3. Balance oxygen atoms by adding H₂O molecules.

The copper half-reaction does not contain O atoms.

Cu(s) → Cu²⁺(aq)

The nitric acid half-reaction shows three O atoms on the left and one on the right, so two water molecules are added to the right side.

HNO₃(aq) → NO(g) + 2 H₂O

Step 4. Balance hydrogen atoms by adding H⁺ions.

The copper half-reaction does not contain H atoms.

Cu(s) → Cu²⁺(aq)

The nitric acid half-reaction shows 1 H atom on the left and four on the right, so 3 hydrogen ions are added to the left side.

HNO₃(aq) + 3 H⁺ → NO(g) + 2 H₂O

Step 5. Balance charge by adding electrons.

The copper half-reaction shows a total charge of 2+ on the right side and 0 on the left side. Adding two electrons to the right side brings charge balance.

Cu(s) → Cu²⁺(aq) + 2 e⁻

The nitric acid half-reaction shows a total charge of 3 on the left side and a total charge of 0 on the right side. Adding three electrons to the left side brings charge balance

HNO₃(aq) + 3 H⁺ + 3 e^– → NO(g) + 2 H₂O

Step 6. Multiply the two half-reactions so the number of electrons in one reaction equals the number of electrons in the other reaction.

To get the same number of electrons in both half reactions, multiple the copper half reaction by 3 and the nitric acid half reaction by 2:

3 Cu(s) → 3 Cu²⁺(aq) + 6 e⁻

2 HNO₃(aq) + 6 H⁺ + 6 e^– → 2 NO(g) + 4 H₂O

Step 7. Add the balanced half-reactions and cancel species that appear on both sides of the equation.

[latex][/latex]

3 Cu(s) + 6 H⁺(aq) + 2 HNO₃(aq) → 3 Cu²⁺(aq) + 2 NO(g) + 4 H₂O(l)

[latex][/latex]

A final check of atom and charge balance confirms the equation is balanced.

Check Your Learning

The reaction above results when using relatively diluted nitric acid. If concentrated nitric acid is used, nitrogen dioxide is produced instead of nitrogen monoxide. Write a balanced equation for this reaction.

Click here for the answer!

Cu(s) + 2 H⁺(aq) + 2 HNO₃(aq) → Cu²⁺(aq) + 2 NO₂(g) + 2 H₂O(l)

Example 17.5 – Balancing Equations for Redox Reactions in Basic Solutions

Write the balanced equation representing reaction between aqueous permanganate ion, MnO₄⁻, and solid chromium(III) hydroxide, Cr(OH)₃, to yield solid manganese(IV) oxide, MnO₂, and aqueous chromate ion, CrO₄²⁻. The reaction takes place in a basic solution.

Solution

Following the steps of the half-reaction method:

Step 1. Write the two half-reactions. Each half-reaction will contain one reactant and one product with one element in common.

oxidation: Cr(OH)₃(s) → CrO₄²⁻(aq)

reduction: MnO₄⁻(aq) → MnO₂(s)

[latex][/latex]

Step 2. Balance all elements except oxygen and hydrogen.

oxidation: Cr(OH)₃(s) → CrO₄²⁻(aq)

reduction: MnO₄⁻(aq) → MnO₂(s)

[latex][/latex]

Step 3. Balance oxygen atoms by adding H₂O molecules.

oxidation: H₂O(l) + Cr(OH)₃(s) → CrO₄²⁻(aq)

reduction: MnO₄⁻(aq) → MnO₂(s) + 2 H₂O(l)

[latex][/latex]

Step 4. Balance hydrogen atoms by adding H⁺ions.

oxidation: H₂O(l) + Cr(OH)₃(s) → CrO₄²⁻(aq) + 5 H⁺(aq)

reduction: 4 H⁺(aq) + MnO₄⁻(aq) → MnO₂(s) + 2 H₂O(l)

[latex][/latex]

Step 5. Balance charge by adding electrons.

oxidation: H₂O(l) + Cr(OH)₃(s) → CrO₄²⁻(aq) + 5 H⁺(aq) + 3 e⁻

reduction: 3 e⁻ + 4 H⁺(aq) + MnO₄⁻(aq) → MnO₂(s) + 2 H₂O(l)

[latex][/latex]

Step 6. Multiply the two half-reactions so the number of electrons in one reaction equals the number of electrons in the other reaction.

This step is not necessary since the number of electrons is already in balance.

[latex][/latex]

Step 7. Add the balanced half-reactions and cancel species that appear on both sides of the equation.

~~H₂O(l)~~ + Cr(OH)₃(s) + ~~3 e⁻~~ + ~~4 H⁺(aq)~~ + MnO₄⁻(aq)

→ CrO₄²⁻(aq) + 1 5 H⁺(aq) + ~~3 e⁻~~ + MnO₂(s) + 1 2 H₂O(l)

[latex][/latex]

Cr(OH)₃(s) + MnO₄⁻(aq) → CrO₄²⁻(aq) + H⁺(aq) + MnO₂(s) + H₂O(l)

[latex][/latex]

If the reaction takes place in a basic medium, add OH⁻ ions the equation obtained in step 7 to neutralize the H⁺ ions (add in equal numbers to both sides of the equation) and simplify.

OH⁻(aq) + Cr(OH)₃(s) + MnO₄⁻(aq)

→ CrO₄²⁻(aq) + H⁺(aq) + OH⁻(aq) + MnO₂(s) + H₂O(l)

[latex][/latex]

OH⁻(aq) + Cr(OH)₃(s) + MnO₄⁻(aq) → CrO₄²⁻(aq) + H₂O(l) + MnO₂(s) + H₂O(l)

[latex][/latex]

OH⁻(aq) + Cr(OH)₃(s) + MnO₄⁻(aq) → CrO₄²⁻(aq) + MnO₂(s) + 2 H₂O(l)

Check Your Learning

Aqueous permanganate ion may also be reduced using aqueous bromide ion, Br⁻, the products of this reaction being solid manganese(IV) oxide and aqueous bromate ion, BrO₃⁻. Write the balanced equation for this reaction occurring in a basic medium.

Click here for the solution!

H₂O(l) + 2 MnO₄⁻(aq) + Br⁻(aq) → 2 MnO₂(s) + BrO₃⁻(aq) + 2 OH⁻(aq)

The requirement of “charge balance” is just a specific type of “mass balance” in which the species in question are electrons. An equation must represent equal numbers of electrons on the reactant and product sides, and so both atoms and charges must be balanced. ↵