9.4  Applying the Ideal Gas Law

Nicole Bouvier-Brown; Saori Shiraki; J. Ryan Hunt; Emily Jarvis

Chapter 9 Gases

9.4 Applying the Ideal Gas Law

Learning Objectives

By the end of this section, you will be able to:

Use the ideal gas law to compute gas densities and molar masses

The study of the chemical behavior of gases was part of the basis of perhaps the most fundamental chemical revolution in history. French nobleman Antoine Lavoisier (Figure 9.19), widely regarded as the “father of modern chemistry,” changed chemistry from a qualitative to a quantitative science through his work with gases. He discovered the law of conservation of matter, discovered the role of oxygen in combustion reactions, determined the composition of air, explained respiration in terms of chemical reactions, and more. He was a casualty of the French Revolution, guillotined in 1794. Of his death, mathematician and astronomer Joseph-Louis Lagrange said, “It took the mob only a moment to remove his head; a century will not suffice to reproduce it.” ^[1] Much of the knowledge we do have about Lavoisier’s contributions is due to his wife, Marie-Anne Paulze Lavoisier, who worked with him in his lab. A trained artist fluent in several languages, she created detailed illustrations of the equipment in his lab, and translated texts from foreign scientists to complement his knowledge. After his execution, she was instrumental in publishing Lavoisier’s major treatise, which unified many concepts of chemistry and laid the groundwork for significant further study.

.

**Figure 9.19** Many credit Antoine Lavoisier and Marie-Anne Paulze Lavoisier with founding “modern chemistry” through their work on gases.

As described in an earlier chapter of this text, we can turn to chemical stoichiometry for answers to many of the questions that ask “How much?” The essential property involved in such use of stoichiometry is the amount of substance, typically measured in moles (n). For gases, molar amount can be derived from convenient experimental measurements of pressure, temperature, and volume. Therefore, these measurements are useful in assessing the stoichiometry of pure gases, gas mixtures, and chemical reactions involving gases. This section will not introduce any new material or ideas, but will provide examples of applications and ways to integrate concepts already discussed.

Gas Density and Molar Mass

The ideal gas law described previously in this chapter relates the properties of pressure P, volume V, temperature T, and molar amount n. This law is universal, relating these properties in identical fashion regardless of the chemical identity of the gas:

P V = n R T

The density d of a gas, on the other hand, is determined by its identity. As described in another chapter of this text, the density of a substance is a characteristic property that may be used to identify the substance.

d = \frac{m}{V}

Rearranging the ideal gas equation to isolate V and substituting into the density equation yields

d = \frac{m}{V} = \frac{m}{\frac{n R T}{P}} = \frac{m P}{n R T} = \frac{m}{n} \frac{P}{R T}

The ratio m/n is the definition of molar mass, M:

M = \frac{m}{n}

The density equation can then be written

d = \frac{m}{n} \frac{P}{R T} = \frac{M P}{R T}

This relation may be used for calculating the densities of gases of known identities at specified values of pressure and temperature as demonstrated in Example 9.11.

Example 9.11 – Measuring Gas Density

What is the density of molecular nitrogen gas at STP?

Solution

The molar mass of molecular nitrogen, N₂, is 28.01 g/mol. Substituting this value along with standard temperature and pressure into the gas density equation yields

[latex]d = \frac {MP}{RT} = \frac {(28.01 \: g/\cancel {mol})(1.00 \: \cancel {atm})}{(0.0821 \: L \cdot \cancel {atm}/ \cancel {mol} \cdot \cancel {K})(273 \: \cancel {K})} = 1.25 \: g/L[/latex]

Check Your Learning

When the identity of a gas is unknown, measurements of the mass, pressure, volume, and temperature of a sample can be used to calculate the molar mass of the gas (a useful property for identification purposes). Combining the ideal gas equation

P V = n R T

and the definition of molar mass

M = \frac{m}{n}

yields the following equation:

M = \frac{m}{n} = \frac{m}{\frac{P V}{R T}} = \frac{m R T}{P V}

Determining the molar mass of a gas via this approach is demonstrated in Example 9.12.

Example 9.12 – Determining the Molecular Formula of a Gas from its Molar Mass and Empirical Formula

Cyclopropane, a gas once used with oxygen as a general anesthetic, is composed of 85.7% carbon and 14.3% hydrogen by mass.

Find the empirical formula.
If 1.56 g of cyclopropane occupies a volume of 1.00 L at 0.984 atm and 50°C, what is the molecular formula for cyclopropane?

Solution

First determine the empirical formula of the gas. Assume 100 g and convert the percentage of each element into grams. Determine the number of moles of carbon and hydrogen in the 100-g sample of cyclopropane. Divide by the smallest number of moles to relate the number of moles of carbon to the number of moles of hydrogen. In the last step, realize that the smallest whole number ratio is the empirical formula:

[latex]85.7 \: g \: C \; \frac {1 \: mol \: C}{12.01 \: g \: C} = 7.14 \: mol \: C[/latex]

[latex]14.3 \: g \: H \; \frac {1 \: mol \: H}{1.01 \: g \: H} = 14.2 \: mol \: H[/latex]

[latex]C = \frac {7.14 \: mol \: C}{7.14 \: mol} = 1.00 \: mol \: C[/latex]

[latex]H = \frac {14.2 \: mol \: H}{7.14 \: mol} = 1.98 \: mol \: H[/latex]

Empirical formula is CH₂ [empirical mass (EM) of 14.03 g/empirical unit].

Next, use the provided values for mass, pressure, temperature and volume to compute the molar mass of the gas:

[latex]M = \frac {mRT}{PV} = \frac {(1.56 \: g)(0.0821 \: \cancel {L} \cdot \cancel {atm}/ mol \cdot \cancel {K})(323 \: \cancel {K})}{(0.984 \: \cancel {atm})(1.00 \: \cancel {L})} = 42.0 \: g/mol[/latex]

Comparing the molar mass to the empirical formula mass shows how many empirical formula units make up a molecule:

[latex]\frac {M}{EM} = \frac {42.0 \: g/mol}{14.0 \: g/mol} = 3.00 = 3[/latex]

The molecular formula is thus derived from the empirical formula by multiplying each of its subscripts by three:

(CH₂)₃= C₃H₆

Check Your Learning

Acetylene, a fuel used welding torches, is composed of 92.3% C and 7.7% H by mass.

Find the empirical formula of acetylene.

CH

[latex]92.3 \: g \: C \; \frac {1 \: mol \: C}{12.01 \: g \: C} = 7.69 \: mol \: C[/latex]

[latex]7.7 \: g \: H \; \frac {1 \: mol \: H}{1.01 \: g \: H} = 7.62 \: mol \: H[/latex]

[latex]C = \frac {7.69 \: mol \: C}{7.62 \: mol} = 1.01 \: mol \: C[/latex]

[latex]H = \frac {7.62 \: mol \: H}{7.62 \: mol} = 1.00 \: mol \: H[/latex]

If 1.10 g of acetylene occupies of volume of 1.00 L at 1.15 atm and 59.5°C, what is the molecular formula for acetylene?

C₂H₂

Use the provided values for mass, pressure, temperature and volume to compute the molar mass of the gas:

[latex]M = \frac {mRT}{PV} = \frac {(1.10 \: g)(0.0821 \: \cancel {L} \cdot \cancel {atm}/ mol \cdot \cancel {K})(332.7 \: \cancel {K})}{(1.15 \: \cancel {atm})(1.00 \: \cancel {L})} = 26.1 \: g/mol[/latex]

Comparing the molar mass to the empirical formula mass shows how many empirical formula units make up a molecule:

[latex]\frac {M}{EM} = \frac {26.1 \: g/mol}{13.02 \: g/mol} = 2.00 = 2[/latex]

The molecular formula is thus derived from the empirical formula by multiplying each of its subscripts by two:

(CH)₂= C₂H₂

Example 9.13 – Determining the Molar Mass of a Volatile Liquid

The approximate molar mass of a volatile liquid can be determined by:

Heating a sample of the liquid in a flask with a tiny hole at the top, which converts the liquid into gas that may escape through the hole
Removing the flask from heat at the instant when the last bit of liquid becomes gas, at which time the flask will be filled with only gaseous sample at ambient pressure
Sealing the flask and permitting the gaseous sample to condense to liquid, and then weighing the flask to determine the sample’s mass (see Figure 9.20)

.

**Figure 9.20** When the volatile liquid in the flask is heated past its boiling point, it becomes gas and drives air out of the flask. At t_l → g, the flask is filled with volatile liquid gas at the same pressure as the atmosphere. If the flask is then cooled to room temperature, the gas condenses and the mass of the gas that filled the flask, and is now liquid, can be measured. (credit: modification of work by Mark Ott)

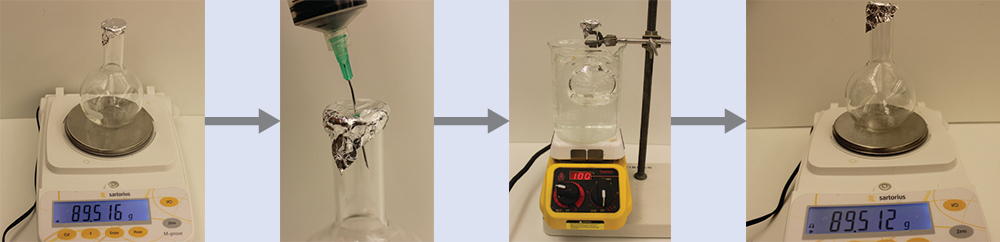
Using this procedure, a sample of chloroform gas weighing 0.494 g is collected in a flask with a volume of 129 cm³ at 99.6°C when the atmospheric pressure is 742.1 mm Hg. What is the approximate molar mass of chloroform?

Solution

Since [latex]M = \frac {m}{n}[/latex] and [latex]n = \frac {PV}{RT}[/latex] substituting and rearranging gives

[latex]M = \frac {m}{n} = \frac {m}{\frac {PV}{RT}} = \frac {mRT}{PV}[/latex]

then

[latex]M = \frac {mRT}{PV} = \frac {(0.494 \: g)(0.08206 \: L \cdot atm/mol \cdot K)(372.8 \: K)}{(0.976 \: atm)(0.129 \: L)} = 120 \: g/mol[/latex]

Check Your Learning

Click here to see how to solve these problems!

To solve for molar mass, make sure to use an appropriate value of R for the provided units of pressure. You must also convert T from °C to K and convert V from mL to L.

[latex]M = \frac {mRT}{PV} = \frac {(3.243 \times 10^{-2} \: g)(8.314 \: kPa \cdot L/mol \cdot K)((550 + 273) \: K)}{(31.89 \: kPa)(0.0560 \: L)} = 124 \: g/mol[/latex]

Comparing the molar mass to the atomic mass of phosphorus shows how many phosphorus atoms make up a molecule:

[latex]\frac {M}{atomic \: mass} = \frac {124 \: g/mol}{30.97 \: g/mol} = 4.00 = 4[/latex]

Media Attributions

Antoine_Laurent_Lavoisier_(1743–1794)_and_His_Wife_(Marie_Anne_Pierrette_Paulze,_1758–1836)_MET_DP-13140-002

“Quotations by Joseph-Louis Lagrange,” last modified February 2006, accessed February 10, 2015, http://www-history.mcs.st-andrews.ac.uk/Quotations/Lagrange.html ↵