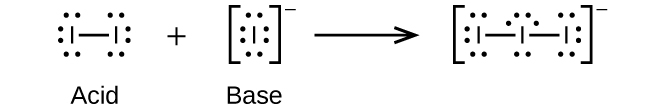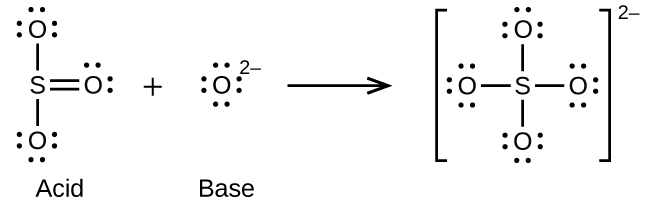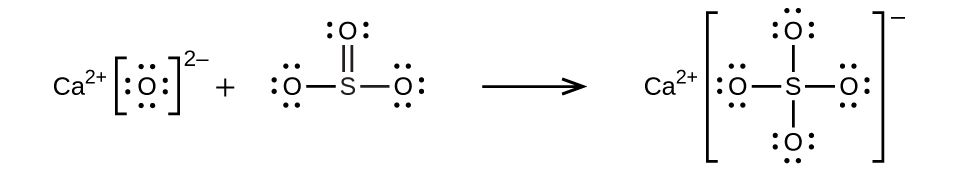Chapter 15 Key

15.1.

(a)

	AgI(s)	⇌	Ag+(aq)	+	I−(aq)
change	(−x)		+x		+x

(b)

	CaCO3(s)	⇌	Ca2+(aq)	+	CO32−(aq)
change	(−x)		+x		+x

(c)

	Mg(OH)2(s)	⇌	Mg2+(aq)	+	2 OH−(aq)
change	(−x)		+x		+2x

(d)

	Mg3(PO4)2(s)	⇌	3 Mg2+(aq)	+	2 PO43−(aq)
change	(−x)		+x		+2/3x

(e)

	Ca5(PO4)3OH(s)	⇌	5 Ca2+(aq)	+	3 PO43−(aq)	+	OH−(aq)
change	(−x)		+5x		+3x		+x

 

15.3.  There is no change. A solid has an activity of 1 whether there is a little or a lot.

 

15.5.  The solubility of silver bromide at the new temperature must be known. Normally the solubility increases and some of the solid silver bromide will dissolve.

 

15.7.  CaF₂, MnCO₃, and ZnS

 

15.9.

(a)  LaF₃(s) ⇌ La³⁺(aq) + 3 F⁻(aq)  K_sp = [La³⁺] [F⁻]³

(b)  CaCO₃(s) ⇌ Ca²⁺(aq) + CO₃​²⁻(aq)  K_sp = [Ca²⁺] [CO₃​²⁻]

(c)  Ag₂SO₄(s) ⇌ 2 Ag⁺(aq) + SO₄​²⁻(aq)  K_sp = [Ag⁺]² [SO₄​²⁻]

(d)  Pb(OH)₃(s) ⇌ Pb²⁺(aq) + 2 OH⁻(aq)  K_sp = [Pb²⁺] [OH⁻]²

 

15.11.  (a)  1.77 × 10^–7 (b)  1.6 × 10^–6 (c)  2.2 × 10^–9 (d)  7.91 × 10^–22

 

15.13.  (a)  2 × 10^–2 M (b)  1.5 × 10^–3 M (c)  2.27 × 10^–9 M (d)  2.2 × 10^–10 M

 

15.15.  CaSO₄∙2H₂O is the most soluble Ca salt in mol/L, and it is also the most soluble Ca salt in g/L.

 

15.17.  4.8 × 10^–3 M = [SO₄​²⁻] = [Ca²⁺]; Since this concentration is higher than 2.60 × 10^–3 M, “gyp” water does not meet the standards.

 

15.19.  Mass (CaSO₄·2H₂O) = 0.72 g/L

 

15.21.

(a)  [Ag⁺] = [I^–] = 1.3 × 10^–5 M

(b)  [Ag⁺] = 2.88 × 10^–2 M,  [SO_4​²⁻] = 1.44 × 10^–2 M

(c)  [Mn²⁺] = 3.7 × 10^–5 M, [OH^–] = 7.4 × 10^–5 M

(d)  [Sr²⁺] = 4.3 × 10^–2 M, [OH^–] = 8.6 × 10^–2 M

(e)  [Mg²⁺] = 1.3 × 10^–4 M,  [OH^–] = 2.6 × 10^–4 M

 

15.23.  (a)  1.45 × 10^–4 (b)  8.2 × 10^–55 (c)  1.35 × 10^–4 (d)  1.18 × 10^–5 (e)  1.08 × 10^–10

 

15.25.  (a)  CaCO₃ does precipitate. (b)  The compound does not precipitate. (c)  The compound does not precipitate. (d)  The compound precipitates.

 

15.27.  3.03 × 10⁻⁷ M

 

15.29.  9.2 × 10⁻¹³ M

 

15.31.  [Ag⁺] = 1.8 × 10^–3 M

 

15.33.  [F⁻] = 6.3 × 10^–4 M

 

15.35.  (a)  2.25 L (b)  7.2 × 10^–7 g

 

15.37.  100% of it is dissolved

 

15.39.

(a)  [Ag⁺] = 6.4 × 10⁻⁹ M ,  [Cl⁻] = 0.025 M.  Check: $\frac{6.4\times {10}^{-9}}{0.025}\times 100\mathrm{\%}=2.6\times {10}^{-5}\mathrm{\%}$, an insignificant change

(b)  [Ca²⁺] = 2.2 × 10⁻⁵ M ,  [F⁻] = 0.0013 M.  Check: $\frac{2.26\times {10}^{-5}}{0.00133}\times 100\mathrm{\%}=1.70\mathrm{\%}$. This value is less than 5% and can be ignored.

(c)  [SO₄​²⁻] = 0.2238 M,  [Ag⁺] = 7.4 × 10^–3 M.  Check: $\frac{3.7\times {10}^{-3}}{0.2238}\times 100\mathrm{\%}=1.64\times {10}^{-2}\mathrm{\%}$.  The condition is satisfied.

(d)  [OH^–] = 2.8 × 10^–3 M,  5.7 × 10⁻¹² M = [Zn²⁺].  Check: $\frac{5.7\times {10}^{-12}}{2.8\times {10}^{-3}}\times 100\mathrm{\%}=2.0\times {10}^{-7}\mathrm{\%}$,  x is less than 5% of [OH^–] and is, therefore, negligible.

 

15.41.

(a)  [Cl^–] = 7.6 × 10⁻³ M.  Check: $\frac{7.6\times {10}^{-3}}{0.025}\times 100\mathrm{\%}=30\mathrm{\%}$.  This value is too large to drop x. Therefore solve by using the quadratic equation:  [Ti⁺] = 3.1 × 10^–2 M,  [Cl^–] = 6.1 × 10^–3

(b)  [Ba²⁺] = 7.7 × 10^–4 M.  Check: $\frac{7.7\times {10}^{-4}}{0.0313}\times 100\mathrm{\%}=2.4\mathrm{\%}$.  Therefore, the condition is satisfied.  [Ba²⁺] = 7.7 × 10^–4 M,  [F^–] = 0.0321 M

(c)  Mg(NO₃)₂ = 0.02444 M,  [C₂O₄​²⁻] = 2.9 × 10⁻⁵ M.  Check: $\frac{2.9\times {10}^{-5}}{0.02444}\times 100\mathrm{\%}=0.12\mathrm{\%}$.  The condition is satisfied; the above value is less than 5%.  [C₂O₄​²⁻] = 2.9 × 10⁻⁵ M,  [Mg²⁺] = 0.0244 M

(d)  [OH^–] = 0.0501 M,  [Ca²⁺] = 3.15 × 10^–3.  Check: $\frac{3.15\times {10}^{-3}}{0.050}\times 100\mathrm{\%}=6.28\mathrm{\%}$.  This value is greater than 5%, so a more exact method, such as successive approximations, must be used.  [Ca²⁺] = 2.8 × 10^–3 M,  [OH^–] = 0.053 × 10^–2 M

 

15.43.  The changes in concentration are greater than 5% and thus exceed the maximum value for disregarding the change.

 

15.45.  (a) Hg_2​²⁺ and Cu²⁺:  add SO₄​²⁻ (b)  SO₄​²⁻ and Cl^–:  add Ba²⁺ (c)  Hg²⁺ and Co²⁺:  add S^2– (d)  Zn²⁺ and Sr²⁺:  add OH^– until [OH^–] = 0.050 M (e) Ba²⁺ and Mg²⁺:  add SO₄​²⁻ (f)  CO₃​²⁻ and OH^–:  add Ba²⁺

 

15.47.  AgI will precipitate first.

 

15.49.  1.5 × 10⁻¹² M

 

15.51.  3.99 kg

 

15.53.  (a)  3.1 × 10^–11 (b)  [Cu²⁺] = 2.6 × 10^–3,  [IO₃​⁻] = 5.3 × 10^–3

 

15.55.  1.8 × 10^–5 g Pb(OH)₂

 

15.57.  Mg(OH)₂(s) ⇌ Mg²⁺ + 2 OH⁻  K_sp = [Mg²⁺] [OH⁻]²

1.23 × 10⁻³ g Mg(OH)₂

 

15.59.  MnCO₃ will form first since it has the smallest K_sp value among these homologous compounds and is therefore the least soluble. MgCO₃∙3H₂O will be the last to precipitate since it has the largest K_sp value and is the most soluble. K_sp value.

 

15.62.  when the amount of solid is so small that a saturated solution is not produced

 

15.64.  1.8 × 10^–5 M

 

15.66.  5 × 10²³

 

15.68.

	Cd(CN)42–	⇌	Cd2+	+	4 CN–
I (M)	0.250		0		0
C (M)	–x		+x		+4x
E (M)	0.250 – x		x		4x

[Cd²⁺] = 9.5 × 10^–5 M,  [CN^–] = 3.8 × 10^–4 M

 

15.70.  [Co³⁺] = 3.0 × 10^–6 M,  [NH₃] = 1.8 × 10^–5 M

 

15.72.  1.3 g

 

15.74.  0.79 g

 

15.76.

(a)

(b)

(c)

(d)

(e)

 

15.78.

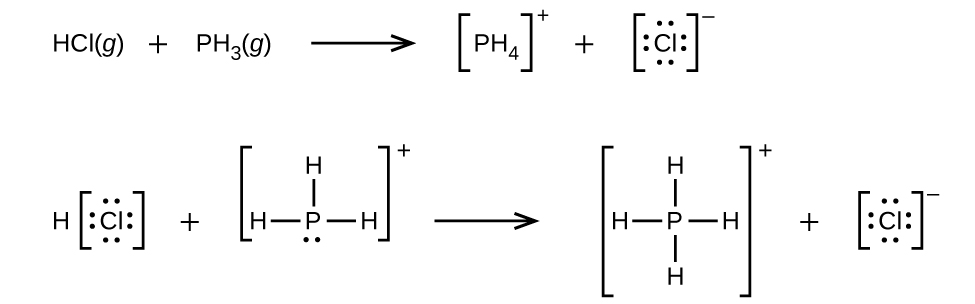
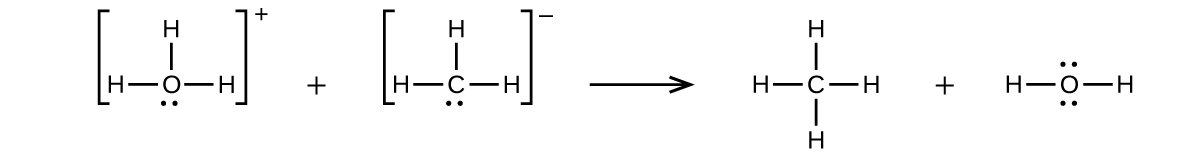
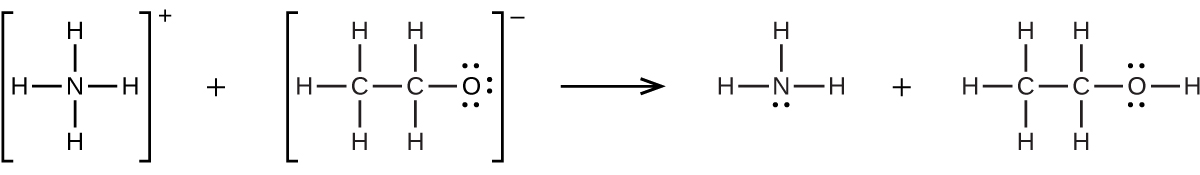
(a)

(b)  H₃O⁺ + CH₃​⁻ → CH₄ + H₂O

(c)  CaO + SO₃ → CaSO₄

(d)  NH₄​⁺ + C₂H₅O⁻ → C₂H₅OH + NH₃

 

15.80.  0.0281 g

 

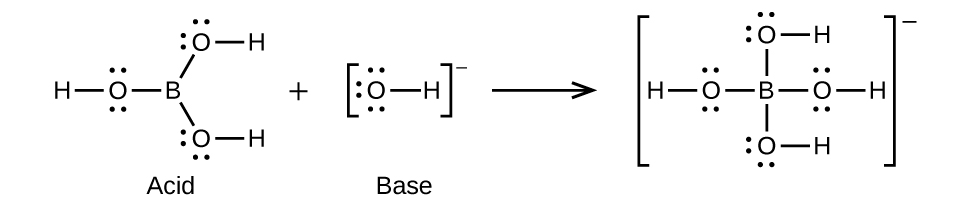
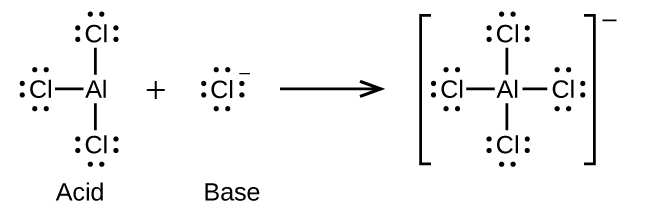
15.82.  HNO₃(l) + HF(l) → H₂NO₃​⁺ + F⁻ HF(l) + BF₃(g) → H⁺ + BF₄⁻

 

15.84.  (a)  H₃BO₃ + H₂O → H₄BO₄​⁻ + H⁺ (b)  The electronic and molecular shapes are the same—both tetrahedral. (c)  The tetrahedral structure is consistent with sp³ hybridization.

 

15.86.  0.014 M

 

15.88.  7.2 × 10^–15 M

 

15.90.  4.4 × 10⁻²² M

 

15.93.  [OH⁻] = 4.5 × 10⁻⁶ [Al³⁺] = 2 × 10^–16 (molar solubility)

 

15.95.  [SO₄​²⁻]=0.049 M [Ba²⁺] = 4.7 × 10^–7 (molar solubility)

 

15.97.  [OH^–] = 7.6 × 10⁻³ M [Pb²⁺] = 2.1 × 10^–11 (molar solubility)

 

15.99.  7.66

 

15.101.

(a)  K_sp = [Mg²⁺][F^–]² = (1.21 × 10^–3)(2 × 1.21 × 10^–3)² = 7.09 × 10^–9

(b)  7.09 × 10^–7 M

(c)  Determine the concentration of Mg²⁺ and F^– that will be present in the final volume using M₁V₁ = M₂V₂.

Compare the value of the ion product  Q_sp with K_sp. If this value is larger than K_sp, precipitation will occur.

(d)  MgF₂ is less soluble at 27°C than at 18°C. Because added heat acts like an added reagent, when it appears on the product side, the Le Châtelier’s principle states that the equilibrium will shift to the reactants’ side to counter the stress. Consequently, less reagent will dissolve. This situation is found in our case. Therefore, the reaction is exothermic.

 

15.103.  BaF₂, Ca₃(PO₄)₂, ZnS; each is a salt of a weak acid, and the [H₃O⁺] from perchloric acid reduces the equilibrium concentration of the anion, thereby increasing the concentration of the cations

 

15.105.  Effect on amount of solid CaHPO₄, [Ca²⁺], [OH^–]: (a)  increase, increase, decrease (b)  decrease, increase, decrease (c)  no effect, no effect, no effect (d)  decrease, increase, decrease (e)  increase, no effect, no effect

15.107. 7.50 mg/L

15.108. (a) 9.9*10^-11 (b) 1.2×10^-9 g Fe(OH)₃ per 100 g solvent (c) In acidic water, the solubility of Fe(OH)₃ increases because H⁺ ions neutralize OH⁻, reducing its concentration and shifting the equilibrium toward dissolving more solid Fe(OH)₃.